Skip to content

Latest commit

 

History

History
86 lines (54 loc) · 2.06 KB

DQM.md

File metadata and controls

86 lines (54 loc) · 2.06 KB
Raw

Table of Contents

  1. Introduction
  2. Technologies Used
  3. Optimization Problem
  4. DQM Model
  5. Results
  6. Setup and Installation
  7. Contributing

Introduction

In this project, I've applied the power of D-Wave's quantum computing to solve a complex optimization problem. This serves both as a portfolio project and a detailed guide for students interested in venturing into the realm of quantum computing.

Technologies Used

  • Python 3.x
  • D-Wave Ocean SDK
  • NetworkX for graph manipulation

Optimization Problem

Objective Function

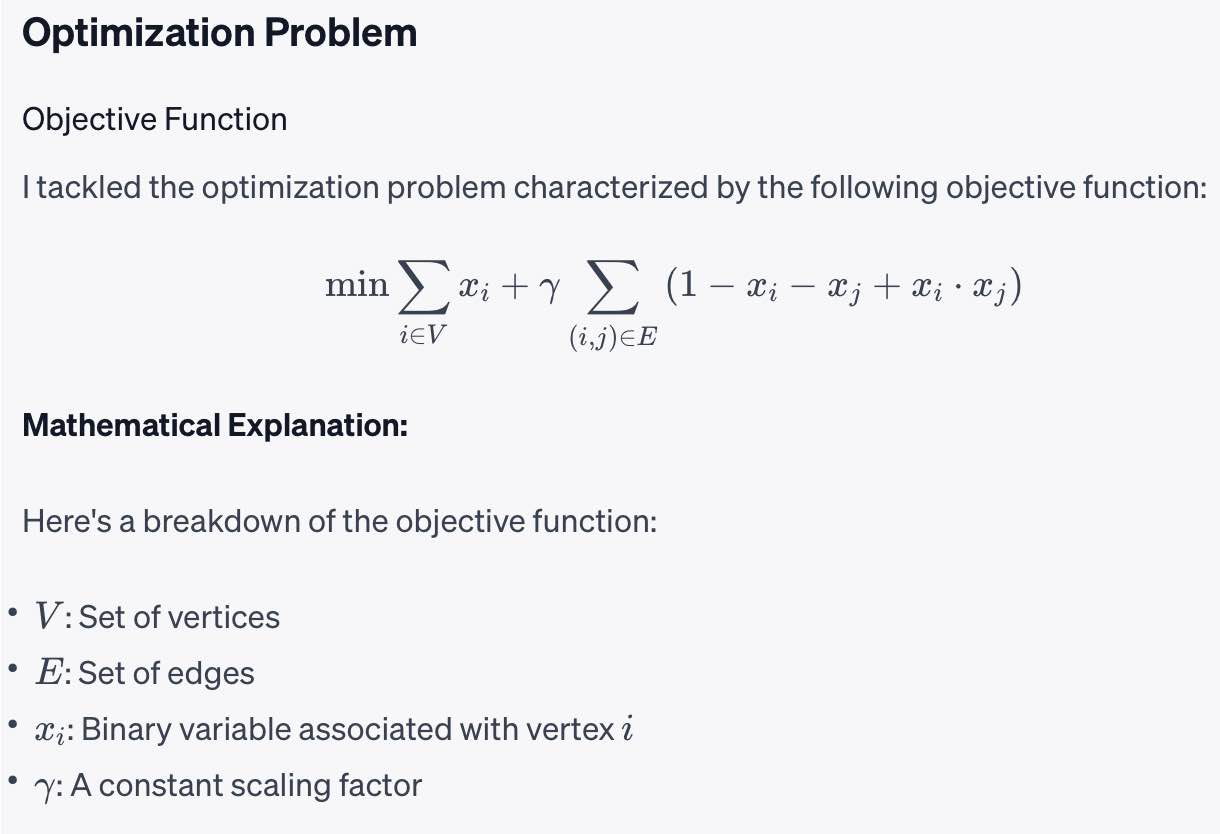
I tackled the optimization problem characterized by the following objective function:

[ \min \sum_{i \in V} x_i+\gamma \sum_{(i, j) \in E}\left(1-x_i-x_j+x_i \cdot x_j\right) ]

Mathematical Explanation:

Here's a breakdown of the objective function:

  • (V): Set of vertices
  • (E): Set of edges
  • (x_i): Binary variable associated with vertex (i)
  • ( \gamma ): A constant scaling factor

DQM Model

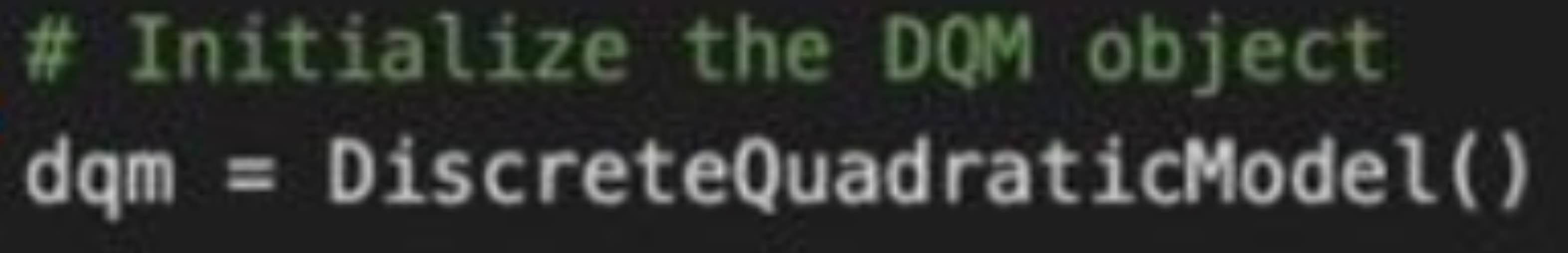
For this project, I've used D-Wave's Discrete Quadratic Model (DQM) to formulate and solve the optimization problem.

# Initialize the DQM object
dqm = DiscreteQuadraticModel()

Code Explanation

  • The Discrete Quadratic Model is initialized using D-Wave's Ocean SDK.
  • Variables and their quadratic interactions are then added to the DQM object.

Results

outcomes, results data visualizations; quantum solutions' efficiency and accuracy.

Setup and Installation

Here are the steps to get this project up and running on your local machine.

# Clone the repository
git clone https://github.com/your-username/your-repo-name.git

# Install dependencies
pip install dwave-ocean-sdk

# Run the code
python your-main-script.py